Geodesics from covariant derivative

                       A geodesic of spacetime is a curve that is straight and uniformly parametrized, as measured in each local Lorentz frame along its way.

If the geosidesic is timelike, then it is a possible wordline for a freely falling particle, and its uniformly ticking parameter λ (called affine parameter) is a multiple of the particule's proper time, λ = κτ + μ.

This definition of geodesic translates into the abstract and coordinate-free language: a geodesic is a curve P(λ) that parallel-transports its tangent vector u = dP/dλ along itself.

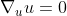
Now defining a coordinate system {x^α(P)), along with basis vectors e_α = ∂/ ∂x^α, we can define the tangent vector u and its components

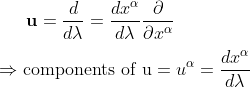
and thus finally  the component version of the abstract geodesic equation definition becomes

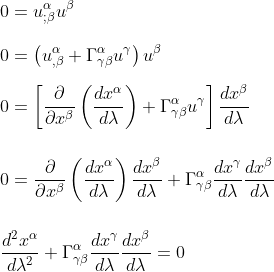
CQFD

This geodesic equation can be solved (in principle) when both initial data x^α and dx^α / dλ have been specified.