In our previous article Gravitational redshift Part III - Experiments, we have mentionned the Pound–Rebka experiment, proposed in 1959 by Robert Pound and his graduate student Glen A. Rebka Jr, to test the gravitational redshift or Einstein effect, predicted as soon as 1907 in Einstein's paper On the relativity Principle and the conclusions drawn from it".
This experiment, as successfull as it was - the result confirmed that the predictions of general relativity were borne out at the 10% level, still had two limitations:
- it only tested gravitational time dilation
- it was not measured with macroscopic clocks

In October 1971, Hafele and Keating flew cesium beam atomic clocks[1] around the world twice on regularly scheduled commercial airline flights, once to the East and once to the West.
In the opening statement of the first of two papers on the subject, the authors refer to the debate surrounding the "twins paradox" and how an experiment with macroscopic clocks might provide an empirical resolution.
In this experiment, both gravitational time dilation and kinematic time dilation are significant - and are in fact of comparable magnitude. Their predicted and measured time dilation effects were as follows:

Let us see how to calculate these relativistic predictions.
Kinematic effects (Special Relativity)
First let us consider as inertial referential the so called ECI (Earth Centered Inertial) with the center of Earth as origin but which does NOT rotate with the Earth[2]. In this non-rotating referential, we will note +v the speed with respect to the earth of the plane flying eastwards and -v the speed with respect to the earth of the plane flying westwards.
Consequently, a plane will fly with the velocity RΩ+v (respectively RΩ-v) with respect to the ECI referential, R being the earth's radius at the equator and Ω the rotational speed of earth. We can use the Newton's addition of speed law there as we ignore the terms in higher order than v2/c2.
The ratio of the proper time interval (as mesured between two beats of the on-board clock) and the time interval as measured in ECI is then given by the usual Lorentz factor, as the consequence of the Transverse Doppler Effect
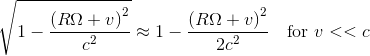
Therefore the relative shift of frequency between a flying clock and another one at rest in the ECI is given by:
This section of the article is only available for our subscribers. Please click here to subscribe to a subscription plan to view this part of the article.

