Geodesic exercise part II: calculation for the surface of the Earth

We will show thereafter that for a surface of a sphere like the Earth:

• - the part of a meridian joining the equator to the north pole is a geodesic
• - a great circle as the equator is also a geodesic.
• - a line of latitude on the top part of the sphere is not a geodesic

 We know from our previous article Geodesic exercise part I: calculation for 2-dimensional Euclidean space that the geodesic equation can be written as

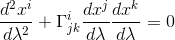
In spherical polar coordinates for a two dimensional surface where r = cste, the index i equals 1 or 2 and x¹ = θ, x² = φ.

We then need the connection coefficients for a surface of a sphere as calculated previously in Christoffel symbol exercise: calculation in polar coordinates part II

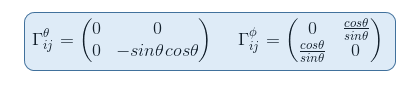
In the geodesic equation, if we replace xⁱ by θ, we have to sum over four elements in the form of Γ^θ_jk.

But the only connection coefficient not null in the form of Γ^θ_jk is Γ^θ_φφ = -sinθ cosθ so that the geodesic equation becomes (i=θ, j=φ, k=φ)

Equation 1

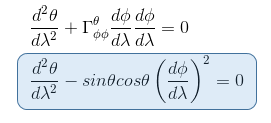
Now we have to replace xⁱ by φ In the geodesic equation, and therefore we have to sum over four elements in the form of Γ^φ_jk.

As the only two connection coefficients in the form of Γ^φ_jk which are not null are Γ^φ_φθ = Γ^φ_θφ = cosθ/sinθ then the geodesic equation becomes (i= φ , j=θ, k=φ and i= φ , j=φ, k=θ ) :

 Equation 2

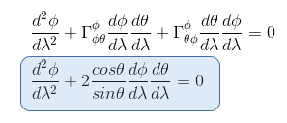
Checking that the meridian is a geodeisc

If we parameterise our meridian by saying that θ = λ where 0 <= λ <= π/2, and φ = 0

then dθ/dλ = 1 => d²θ/dλ² = 0   also as φ = 0, then dφ/dλ = d²φ/dλ² = 0

So equation 1 becomes 0 - sinθcosθ x 0 = 0 so 0 = 0 which holds true

In the same way, equation 2 becomes 0 + 2(cosθ/sinθ) x 1 x 0 = 0 <=> 0 = 0 which is verified

Both equations 1 and 2 are true (left-hand side equals the right hand side), therefore they satisfy the geodesic equations, meaning we have shown that the meridian is a geodesic.

Checking that the equator is a geodeisc

If we parameterise our equator by saying that θ = π/2  and φ = λ where 0 <= λ <= 2π

then dφ/dλ = 1 => d²φ/dλ² = 0   also as θ = π/2, then dθ/dλ = d²θ/dλ² = 0  and cosθ = cos (π/2) = 0 sinθ = sin(π/2)=1

So equation 1 becomes 0 - 1 x 0 x 1² = 0 so 0 = 0 which holds true

In the same way, equation 2 becomes 0 + 2 x 0 x 0 x 1 = 0 <=> 0 = 0 which is verified

Because  equations 1 and 2 are true (left-hand side equals the right hand side), therefore they satisfy the geodesic equations, meaning we have shown that the equator is a geodesic.

Checking that a top latitude line is not a geodeisc

Let's take now the example of a circle on the top part of the sphere defined by θ = π/4 and φ = λ where 0 <= λ <= 2π

Then dφ/dλ = 1 => d²φ/dλ² = 0   also as θ = π/4, then dθ/dλ = d²θ/dλ² = 0  and cosθ = cos (π/4) =  sinθ = sin(π/4)= √ 2

So equation 1 becomes 0 - √ 2 x √ 2 x 1² = -2 ! = 0 (not true!)

Equation  2 becomes 0 + 2 x 1 x 0 = 0 <=> 0 = 0 which is verified

As equation 1 is not verified even if equation 2 Is verified, it means this top circle of latitude is a not geodesic.