Suppose that Alice has some unknown qubit that she wants to send to Bob. She can not measure the qubit to see what it was, for that this would inherently modify the qubit by collapsing its state. This is obviously not what we want.
Why don’t we just make a copy of it? Unfortunately this is what the no-cloning theorem forbids. Formally, it states that we cannot clone arbitrary quantum states by unitary evolution.
Theorem: It is impossible to create an identical copy of an arbitrary unknown quantum state.
Proof (by contradiction):
Suppose that there exists a unitary operator C that copies an arbitrary unknown q-state. That is C is a two-qubit operator that copies or clones the first qubit into the second qubit
Since the theorem is about the ability of C to clone any arbitrary state, let’s write down the copy for two random states |Ψ1> and |Ψ2> that should be copied into the state |ρ>
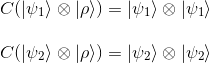
Taking the inner product of these two expressions we get:
This section of the article is only available for our subscribers. Please click here to subscribe to a subscription plan to view this part of the article.